The Bernoulli Equation
Applying the Bernoulli equation, often referred to as the Bernoulli principle, is only applicable in a tube, like a pipe. It is never applicable for an unconfined fluid like the air over a wing, a hurricane taking off a roof, or the confinement of a ping pong ball in a jet of air. If it weren’t for the abundance of misapplications of the Bernoulli equation, the name Coanda would be on people’s lips, not Bernoulli.
The Bernoulli equation is a statement of the conservation of energy. That means that energy cannot be added or subtracted from the system. Even then, it is only an approximation due to energy losses to viscosity and friction with the walls of a tube. It is applicable for the venturi and for confined fluids that change height in a gravitational field. We will discuss neither. As Brutus said about Caesar, we are here to bury Bernoulli, not to praise him.
We will start with the simplest statement of the energy of a system. The total energy, Et, of a system equals the potential energy, Ep, plus the kinetic energy, Ek. Potential energy is the stored energy that an object possesses due to its position, arrangement, or state that can be converted into another form of energy. Examples are a compressed spring, the pressure in a balloon, or a rock on top of a high ledge.
Kinetic energy is the energy of an object due to motion. This is the energy in a fast-moving car or bullet. The Kinetic energy of an object is ½ mv2, where m is the mass of the object and v is its velocity. The simplest statement of the energy of an object is
Et = Ep + Ek. (1)
Now, the energy of the air in a balloon is equal to the pressure times the volume. Reversing the order, energy divided by volume is pressure. So, for a system where volume is constant, as in an incompressible gas, the energy in the gas is equal to:
Et/V = Ep/V + Ek/V (2)
Or
Pt = Ps +Pd (3)
V is the volume, Pt is the total pressure, Ps is the gas’s static pressure, and Pd is the kinetic or dynamic pressure. In physics, Pp is written Ps, which is the static pressure around you in the room or what one would experience riding along in flowing air. Pk is written as Pd, which is the dynamic pressure. It is not a pressure but has the dimensions of pressure and is directional. It is the pressure one feels when the wind blows against you, but not when it is blowing away.
As most of us have been told, the Bernoulli equation is a statement of the conservation of energy. That means that the total energy, in this instance, the total pressure, is a constant. Rewriting equation (3) with Pt a constant, C, we have the Bernoulli equation:
C = Ps + Pd. (4)
The restrictions on this equation are:
- The fluid must be incompressible.
- All must be in equilibrium, such as a constant speed and pressure
- No energy can be added or subtracted from the system.
In this discussion, the altitude of the flow is constant.
An important point is that an unconfined flowing fluid will always have the static pressure of the surrounding environment. There is no membrane separating the static pressure of the flowing fluid from the static pressure of the environment. In the case of lift on a wing, the lower pressure of the air flowing over the wing is not due to the motion of the air but to the lowering of the pressure, drawing a large quantity of air perpendicular to the flight path, producing lift.
A simple example of fast-moving air not having a lower pressure is the static port on an airplane. Almost all airplanes have a small hole on the side of the fuselage called the static port. (In a few older airplanes, the static port is on the side of the pitot tube.) The purpose of the static port is to provide air pressure information to the altimeter and other flight instruments. The altimeter can measure the change in altitude by extremely small pressure changes. When the airplane is sitting quietly at the tie-down, the pilot adjusts the altimeter for the change in barometric pressure so the altimeter gives the correct altitude of the airport. For a single-engine airplane, during the pre-flight runup, the propeller accelerates air at more than 100 knots over the static port without any change in indicated altitude. In flight, the static port may be moving through the air at twice that speed, again giving the correct altitude. So, in short, the pressure at the static port provides the correct pressure if the air is still flowing across the port or the port is rapidly moving through the air.
Bernoulli and Aeronautics
Anyone who argues the Bernoulli explanation of lift will state that aeronautical engineers (AE) use the Bernoulli equation in their calculations. We’ve even been told this by AEs themselves. So, let’s get this straight.
The Bernoulli equation, or something like it, is used in some simulations. But since energy must be held constant, it is only applicable to simulations of 2-D or infinite wings. Two-dimensional wings and infinite wings produce lift without doing work, so they do not violate the restrictions above. But you probably won’t see them on airplanes in the sky.
Now comes the simulations of 3-D wings, which require work to produce lift. The Bernoulli equation is not applicable here, though they think they are using it. In the simulation, energy is lost due to induced drag that is not corrected for. The wing is slowing down. They are violating numbers (2) and (3) of the restrictions in the Bernoulli equation above. They get around this by taking a snapshot of the system. They catch it at a moment in time. Then they apply equation (3) above, not the Bernoulli equation (4). That may be correct, but they are certainly not applying the Bernoulli equation.
The humorous thing is that they don’t know that they are doing this. They don’t realize that they’re taking data on a wing that is losing energy, is slowing down, and is not in equilibrium.
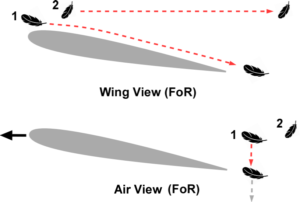
Let’s assume that a high-flying goose loses two downy feathers. As they slowly drift down, an airplane passes its wing just under one of the feathers. See Figure 1. The wing passes, say 1 foot, under feather #1. Feather #2 is approximately the same height as the first feather, but far enough away not to be disturbed by the wing. The “wing view” or the pilot’s view shows the first feather streaking back and coming off at a downward angle. That’s the downwash. Feather #2 streaks back at the same speed but at a constant altitude. In this frame of reference, one can imagine applying the Bernoulli principle. After all, it is the wind-tunnel frame of reference.
Now let’s look at the same event, riding along with the air in a hot-air balloon. We have shown that an airplane flies in still air. So is the balloon. Feather #1 goes straight down as we understand it should. The air is accelerated perpendicular to the flight path to produce an equal and opposite force. Feather #2 is, of course, stationary. Since no air flows over the wing, the Bernoulli principle cannot be applied.
So, there we have it! The Bernoulli explanation of lift is “rest-frame dependent”. But the physics of lift cannot be rest-frame dependent. The physics of the interaction of the wing and the air does not depend on the observer. The Bernoulli equation may be of some use in simulations, but it has nothing to do with the physics of lift. No more needs to be said.








