Introduction
This web page explains how a wing develops lift. It will help you replace your favorite lift “plausible falsehoods”, i.e., myths, with real physics. Later we will explain the details of lift and the importance of power. We’ve learned that the old, tightly held misconceptions must be put aside first or there will be a tendency to try to incorporate the old ideas into the new. Then with time, the new ideas become less clear and the old creeps back in.
If you are reading this web page without reading the web pages discussing misconceptions 1, 2, and 3, we’ll provide a brief recap of the misconceptions as dogma. But we hope you have addressed flight in an orderly way and that what immediately follows is not entirely new to you.
- Higher airspeed does not mean lower static air pressure – Misconception #1 – Bernoulli Misconceptions. This misconception is the cause of the most confusion. Unconfined high-speed air has the same static pressure as the surrounding environment. The Bernoulli equation which relates pressure and speed of a fluid, is only valid in a pipe – a confined space – and certainly not on the top of a wing.
- The shape of the wing has nothing to do with lift production. It does affect cruise efficiency and stall characteristics. Misconception #2 – Shape of the Wing. This is another misconception that has led to confusion. Most believe that lift is related to the shape of the wing. However, in principle, all wings can fly upside down.
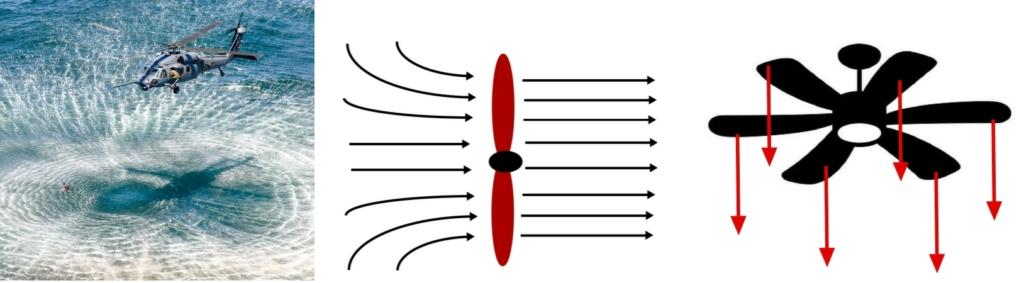
- Look at Figure 1 of a helicopter, a propeller, and a ceiling fan. All are legitimate wings that accelerate air perpendicular to their plane of rotation. The Wright brothers developed the modern propeller by realizing it was just a rotating wing.
Before we discuss the physics of flight, let’s take a quick refresher on Newton’s three laws. We’ll discuss Newton’s First and Third Laws of Motion and then Newton’s Second Law of Motion.
Newton’s Laws of Motion
Newton’s first and third laws
Newton’s first law states: that an object at rest will remain at rest, or if in motion, will remain in straight-line motion unless acted on by an external force.
A simple example is a shopping cart. It is stationary until one gives it a push (force) and travels in a straight line until a force is put on it to stop. Another example is gravity. It bends down a stream of water from a garden hose.
Newton’s third law states that if object A puts a force on object B, then object B puts an equal and opposite force on object A That is, for every force there is an equal and opposite force. When you pushed on the shopping cart, you felt the shopping cart press on your hands.
A few other examples of Newton’s third law: You may be sitting on a chair reading this. Your weight presses on the chair and the chair presses on you. Or, take a book lying on a table. The weight of the book presses down on the table and the table presses up on the book with the same force.
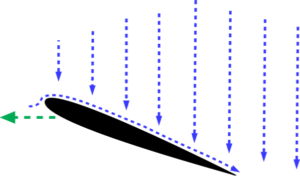
One problem with the Bernoulli explanation of lift – see Figure 2 – is that there is no pairing of opposite forces. Only two forces are ever shown on a wing: lift and weight. Remember that gravity (which is a force) is the attraction of two objects. So, in level flight, the airplane’s weight is paired with the equal and opposite attraction of the earth by the airplane, just as the earth pulls on the moon and the moon pulls on the earth. The second paired forces are the lift on the wing and the force on the air. Because lift is straight up, Newton’s third law requires the force on the air to be straight down (in straight and level flight) and equal to the airplane’s weight.
Newton’s second law
Newton’s second law is more complicated. It states that the force is equal to the momentum’s rate of change.
The momentum of an object is its mass times its velocity and is one of those quantities that nature wants to conserve like energy and angular momentum. Take the example of a car on the highway. It has a certain weight (mass) and a certain speed thus a certain momentum. If its speed is doubled, its momentum will double. The increase in speed, and therefore momentum, requires a force.
So, the force on an object is either equal to the rate of change of the mass or the rate of change of the velocity (acceleration). With few exceptions, Newton’s second law is written F=ma, where “m” is the mass and “a” is the acceleration of an object.
To calculate the force (thrust) of a rocket, the thrust is equal to the rate of change of the mass in the exhaust times the velocity of that exhaust. This is written F=Δmv where Δm is the rate of change of the mass. The rate of change in mass could be Kg per second or the rate mass is expelled in the exhaust.
The force caused by the rocket’s exhaust is similar to the recoil force of a hose. You can demonstrate this for yourself. Hold a hose in one hand then turn the water on full blast. You’ll observe the water rapidly flowing from the hose and feel the opposite force forcing your hand away from the water stream. This is the form of Newton’s second law that is applicable to lift of a wing. Another good example is the water jet ride.
Lift on a Wing
We know from Misconception #3 – Airflow Direction, that air is accelerated perpendicular to the flight path, to produce the lift on a wing. This is illustrated in Figure 3. Thus, according to Newton’s first law, there must be a force on the air because the air moves. Newton’s third law states that there must be an equal and opposite force on the wing, i.e., lift. Newton’s second law says that force is equal to the change in momentum of the air, which is the rate at which the air is accelerated times the velocity of the air.
Thus, the lift on a wing is equal to the rate at which air is accelerated times the speed of that air. It’s as simple as that.
So, there we have lift for all wings in flight. The above statement is the explanation of lift. It is simple, elegant, and doesn’t involve the shape of the wing, the Bernoulli principle, circulation theory, or any other well-meaning yet false hypothesis.
How much air?
A back-of-the-envelope calculation for a Cessna 172 at cruise speeds accelerates several tons of air per second [1]. This air is accelerated from well over 20 feet above the wing. A jumbo jet in cruise flight develops a downwash that accelerates air on the order of its weight per second. A wing’s secret to efficiency is that it accelerates a lot of air at a very low velocity. This is discussed in more detail in Lift Details.
The Bernoulli view of lift falsely states that the air flows faster over the top of the wing, causing a lowering of the pressure. Even if that were true, the problem with this hypothesis is that the wing doesn’t interact with nearly enough air to produce the lift. In fact, in a stall, the air that flows over the wing does not even make it to the trailing edge of the wing. And, as pointed out in Misconception #3, all airplanes, neglecting turbulence, fly in still air, and it is the wing that is moving through the air, not the air moving over the wing.
In Lift Details, we discuss how lift changes with changes in speed, air density, and angle of attack. These topics get a little technical, but there is no way around it without completely dumbing down the subject.
Take Away
- A wing develops lift by accelerating air perpendicular to the flight path.
- Lift is equal to the rate at which air is accelerated times the velocity of that air.
- A wing flies in still air. Thus, the wing moves through the air, and not that air moves over the wing.
References
- David F. Anderson and Scott Eberhardt. Understanding Flight, 2nd ed. (McGraw-Hill, 2010).